Tunneling Beneath the 4He Fragmentation Energy

J. Condensed Matter Nucl. Sci. 4 (2011) 241–255
Research Article

Tunneling Beneath the 4He∗ Fragmentation Energy
Andrew Meulenberg ∗
National Advanced IPv6 Centre, Universiti Sains Malaysia, 11800 Penang, Malaysia

Krityunjai P. Sinha †
Department of Physics, Indian Institute of Science, Bangalore 560012, India

Abstract

The repulsive Coulomb barrier between deuterium nuclei is reduced in length and height by a catalytic mechanism involving optical
phonons and electric fields in a lattice. If this mechanism induces the formation of D− D+ pairs, the tightly bound and energetic
electron pair (a “lochon” in the D− ion) becomes an attractive force between the nuclei. The lattice constraints and slow collision
processes force the ions into a near 1-D configuration within the lattice that deepens the electron ground-state potential well. This
permits the electron pair to remain closely bound to one deuteron and to do work in bringing the D− D+ pair together. The work
done reduces the nuclear-mass deficit (transferring it to electron kinetic and field energy) and that, along with the reduced Coulomb
repulsion of the nuclear protons, brings down the helium nuclear-energy levels of the fusing pair and raises the 4He fragmentation
level. The proposed model accounts for the observations in condensed-matter nuclear science (CMNS) of excess heat (in both p–p and
d–d reactions) and the differing observations (or for the absence) of tritium, 3He, neutrons, and 4He. The variation (unpredictability)
of results (inherent in the many experiments) and evidence for transmutation, heretofore stumbling blocks to acceptability of CMNS,
is now perhaps a validation of its existence. All major observed CMNS processes are addressed by the model.

© 2011 ISCMNS. All rights reserved.

Keywords: CMNS, Catalyst, Deuterium, LENR, Phonon-assisted, Tight-binding

PACS: 21.10.Dr Binding energies and masses, 25.10.+s Nuclear reactions involving few nucleon systems, 25.30.-c Lepton-induced
reactions, 25.60.Pj Fusion reactions, 25.90.+k Other topics in nuclear reactions: specific reactions, 27.10.+hA ≤ 5

1. Introduction

The Fleischman–Pons effect, observed in a palladium lattice electrolytically loaded with deuterium, is a release of
energy greatly in excess of that possible by chemical means. The explanation proposed for the effect (a low-kineticenergy
nuclear-fusion of two-deuterium atoms) and the experimental results violated three known aspects of deuteron
(d–d) fusion in Nuclear Physics. These are:
∗E-mail: mules333@gmail.com
†E-mail: kpsinha@gmail.com
© 2011 ISCMNS. All rights reserved.
242

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

1. Ultra-low probability of reaction: Tunneling of deuterons must occur through a much longer and higher internuclear
Coulomb barrier than could be penetrated at the rates required to provide the excess heat observed.

2. Fragmentation and fragmentation ratio: The high fragmentation rates that would result from conventional
penetration of the d–d Coulomb barrier and the formation of an excited 4He nucleus (4He∗) are not observed.
The fragmentation ratio, n:p, from D+D ⇒ 3He+n and D+D ⇒T + p is expected to be ∼50:50 (neutron +
3He and proton + 3H emission are considered to be nuclear “ash”).

3. Decay paths from excited states of 4He: No energetic gamma rays from direct de-excitation of fused D–D nuclei
to the 4He ground state (or products from another mechanism for delivering nuclear energy to the lattice) are
observed.

Furthermore, subsequent low-energy-nuclear-reaction (LENR) research has often failed to reproduce the effect, has
produced a large variety of (instead of consistent) results, and has provided strong evidence of changes in elemental
composition (transmutation [1]) that is not possible from simple fusion reactions. Various models have been proposed
to support the multiple experimental results and to overcome the arguments against a nuclear process. Few have been
able to answer all of these challenges. None has yet been accepted, even by a large number of LENR researchers.
The present authors have developed a model ([2–5] reviewed briefly below) to solve the first of these problems using
accepted physics of the solid state for the greater stability (perhaps cyclic) of charged sites relative to uncharged sites
in some crystal lattices [6]. This knowledge was applied to the PdD lattice to suggest paired electrons in the same state
(i.e., s-orbit in a D−ion) becoming a local-charged Boson – the “lochon” – and the resulting D− D+ ion pairs being
attractive rather than being repelled by the normal d–d Coulomb barrier [7]. The present work shows how extension
of this lochon model supports the reduction in total energy of the colliding nucleons, and thereby explains most of the
other experimental results observed in the field (including items 2 and 3 above).
The next sections describe the Lochon model, its extension, and its implications - from the PdD lattice sites, to the
d–d near-field, and to the nuclear-interaction region. The nuclear issues are then addressed along with the mechanisms
whereby the extended model is able to explain even the unexpected observations of LENR. Two appendices provide
details of the extended-lochon process and address some applicable semi-classical physical-property issues of electrons
and orbits that are never mentioned in quantum-mechanics texts and papers. A final appendix mentions a mathematical
construction for a deep, bosonic, atomic orbital that might be verified by the experimental LENR results and the
extended-lochon model.

2. Lochon Model
We have previously presented a model whereby the repulsive Coulomb barrier between hydrogen (deuterium) nuclei is
reduced in height and length, perhaps by orders of magnitude [2]. This lochon-catalyzed-fusion mechanism, involving
longitudinal optical phonons and local electric fields (internally or externally generated) in a crystal lattice that induce
the formation of D− D+ pairs, increases the low-energy-tunneling probability by more than 100 orders of magnitude
relative to that predicted from models based on multi-MeV deuteron-beam experiments [2].
We are dealing with a composite system (PdDx ) where two types of atoms or ions exist. Thus the primary lattice
is palladium and the sub-lattice is hydrogen (deuterium). However, for our situation, the most relevant modes may be
the interface phonon modes. The solutions to the quantum mechanical equations representing this system correspond
to localized optical-phonon modes near the interface. The optical phonon mode of interest has the deuterons, beating
against each other at the sub-lattice phonon frequency (∼ 1014/s). The reduced dimensionality at an interface, or at a
linear defect, deepens the potential well, thus creating higher-frequency phonon modes. These higher-energy modes
create resonant electrostatic fields that are strongly coupled with the electrons confined in the layer. The addition of
laser stimulation [2] increases the resonant phonon-field amplitudes, the D− D+ pair production, and the energy at
which the local Coulomb barrier is encountered. This external resonant field can be adjusted to selectively stimulate the

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

243
higher-frequency modes and the counter moving deuteron modes. Phonon-induced electron pairing in a deepened well
provides a basis for filling the deuteriums electron ground-state s-orbital that further localizes the pair [8] and binds it
to a deuteron during its resonant oscillations within a lattice site.
The proposed electron pair would result in sub-lattice D− D+ pairs [9] and become more than strong screening; it
would become an attractive force between the nuclei [5]. Thus, as the deuterons approach each other closely and deeply
penetrate the D− distributed-electron Coulomb field, the residual proton-proton (or d–d) Coulomb barrier is found to
be much reduced, in height as well as in length. With the resulting enhanced barrier-penetration probability, the energy
level of nuclei with reasonable tunneling probability may drop from the multi-100 keV range down into the many eV
range, thus leading to low-energy nuclear reactions.
In this early version of our model (whichwas only concerned with the barrier penetration problem), normal tunneling
of deuterons is into resonant excited 4He∗ nuclear states at energy levels above the fragmentation levels [10]. Thus, even
if the Coulomb barrier is overcome by the tightly-bound electron pair and fusion is possible, the major observations
of LENR (e.g. more heat than accounted for by neutron producing reactions) are not possible. This requirement of
tunneling into states above fragmentation is dictated by the mass deficit (Q =23.8 MeV [11]) between the two colliding
D atoms and the resultant 4He atom. Therefore, even with no incident kinetic energy, a deuteron pair has too much mass
energy to tunnel beneath the fragmentation levels [12] at 20.6 and 19.8 MeV above the 4He ground state. Nevertheless,
the model was successful in providing a mechanism (with mathematical predictions of fusion rates consistent with
observation) to counter arguments against LENR (item 1 above).

3. Extended Lochon Model
In a more detailed study of the lochon model, new and unexpected effects are found. Just as slow-motion photography
can reveal unsuspected processes, the study of low-energy collisions between charged particles (i.e., eV compared
to MeV) has provided some surprises. It is the non-equilibrium conditions of a “slow” D− D+ collision in a linear
sub-lattice or lattice defect that provides a possible answer to the nuclear ash problem of CMNS (item 2 above). The
deuteron motion is slow relative to the bound-electron motion. This distinction allows us to separate their actions and
use the Born–Oppenheimer approximation to solve for the electron quasi-steady-state parameters, step-by-step, as the
D− D+ pair approach each other over the lattice barrier separating them (and afterwards, Appendix A).
The D–D molecular potential well deepens as an extended D2 molecule is being confined to two dimensions
(Fig. 1) [13] by the lattice and Coulomb fields. During this process, the electron potential well also deepens (by
reduced dimensionality and by the quadratic Stark effect from the confining lattice electric fields). A deepening of the
electron potential wells does not necessarily mean that the electrons go deeper into the well; they must lose total energy
to do that (although they gain kinetic energy in the process). However, being in a ground state (l = 0, with no lower
energy states having angular momentum) they cannot directly radiate photons or generate phonons. (This would be a
highly forbidden transition, since both processes require a change in angular momentum of _l = 1). Because of the
differences in resonant frequencies involved, indirect radiation of energy (via near-field coupling with lattice phonons)
is slow. Nevertheless, in a critical concept provided by Tom Barnard [14], a mechanism is provided whereby these
electrons may dissipate energy as the separation of the D–D+ pair is reduced.
Since work is done by the electrons during this movement (they transfer energy to the deuterons), they fall further
into the deuterons deepening Coulomb potential well. Part of this work is a result of “lochon drag,” which is analogous
to phonon drag effects [15] on electrons in a lattice. Being deeper in the well means that one or both of the paired
electrons is less likely to transfer to the potential well of the other deuteron during the process. This is a critical
assumption of the model [16].
Since this electron pairing is a possible state, quantum mechanics can calculate its energy relative to that of zero
or single-electron occupancy [6]. However, the probability of being in that state depends on the energy of the electron
244

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

Figure 1. Molecular potential well for 3-D, 2-D, and 1-Dimension hydrogen-molecule configurations using atomic units (R = 0.53 Å and
V (R) = 27.2 eV). The PdH lattice represented here (in planar view) includes an interface at which the tri-linear H sub-lattice is reduced in
dimensionality.
pair and the local Fermi levels and electric fields; and these in turn depend on the recent history of the D− D+ pair.

This is thus a time-dependent calculation. A consequence of the electron pairing in this model is that a portion of the
electric-field energy of the D− D+ pair can be converted into work to overcome the lattice barrier (many-eV range)
and the d–d Coulomb barrier (keV to MeV range) as they get closer. The effect of unpaired electrons, relative to that
of paired electron(s), for LENR has been explored and found to be of little consequence.

3.1. Slow interactions
Nuclear physics collision experiments are diabatic. The equilibrium electron-energy levels do not have time to change
before the event is over. On the other hand, the critical portion of the D−D+ interaction in a lattice is adiabatic.
The “slow” motion of the converging deuterons allows hundreds of electron orbital cycles to occur during each step
and therefore allows the electrons time to experience and respond to the changing fields. Furthermore, nuclear physics
experiments are unlikely to see any of this D−D+ pairing effect unless the target deuterons have a reasonable probability
of being in the negative ion state at the moment of collision. On the other hand, under the influence of lattice opticalphonon
motion, that moment may be precisely when this state is most likely. A deuteron-beam experiment would have
only a low statistical probability of involving a negative deuterium ion. Also, the mathematical interpretation of nuclear
physics experiments under its low-energy (e.g. ∼5 keV) collision conditions would only indicate a higher Coulomb
screening [17]. This interpretation may be appropriate for a deuteron with bound electrons. However, there are aspects
of the LENR experimental results and of this model that any nuclear physics experiment is unlikely to see.
As the bound electrons move deeper into the D− Coulomb potential well, the cancellation of external electric fields
(of the electron and proton) reduces the external field energy (potential energy) and therefore the “size” and (initially

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

245
very slightly) the mass of the charged particles [18]. Since energy is conserved, part of this loss in field energy goes
into the increased kinetic energy of the electrons (Te), part into the increased kinetic energy of the deuterons (Td), and
part into increasing the inter electron–deuteron electric fields as the oppositely charged particles move closer together
(Appendix A). However, just as the electrons do work in bringing the deuterons together, the deuteron and electron pairs
must have work done on them to move them closer together. Thus, there are compensating field contributions from
the e–e and d–d interactions as well. The net result of this 3 or 4-body interaction is that the tightly-bound, energetic,
electrons (lochon or e#s) and deuterons lose total energy (they have more KE, but have moved even deeper into the
local Coulomb wells).With proximity, the D−D+ attractive Coulomb potential grows, and collapse of the D−D+ pair
is accelerated. Nevertheless, the initial energy expended in overcoming the lattice barrier keeps the D−D+ pair in
the adiabatic regime (at low velocity) until the pair gets very close (∼10 pm?). As the pair gets still closer together,
the D−D+ Coulomb potential dominates the lattice barrier and the closing velocity increases. However, during this
same period, the electron kinetic energy also increases greatly so that the relative velocities (electron to deuteron) are
maintained in the adiabatic regime.
The electron’s kinetic-energy-increase and their movement deeper into the Coulomb well about the deuteron causes
them and their orbit to “shrink” (Appendices B and A). The electron deBroglie wavelength decreases with increased
velocity and, as it spirals in, its external-field energy is further “cancelled” by that of the proton. With an increase in
energy from the Te = multi-eV range of electrons in sub-lattice deuterium atoms to the keV range of a bound electron
as it approaches the deuteron, the deBroglie wavelength is reduced by an order of magnitude. As the e# kinetic energy
increases to the 100 keV range, the wavelength drops by 10×again and approaches that of the electron Compton radius.
However, by this time, the peak electron field (and therefore the electron center of “mass”) has shifted to within 100s
of Fermi of the nucleus.
3.2. Electron–proton interaction in the 1–10 fm range
As the electron moves even closer to the nucleus (within 10 fm), its instantaneous kinetic energy continues to increase;
but, it begins to lose its identity and may no longer be considered a separate entity (Fig. 2). The e#–p pair has become
a relativistic rotating dipole field (monopole + quadrupole field, if two bound electrons are present). The cancelled
charge far-field energy has been replaced by near-field, electromagnetic and relativistic-mass energy. In support of this
proposed semi-classical kinematic model for the (brief?) existence of tightly bound electron pairs, recent mathematical
work [19] uses a solution of the Klein–Gordon equation to indicate the possibility of a deep energy level [20] in the
hydrogen atom (Appendix C) for a charged boson, such as the lochon.

Figure 2a shows the consequences of electron–proton proximity on the electric potential (assuming stationary
charges) as an example of the size/shape change effect mentioned above and in Appendix B. (Resolution of Fig. 2b is
insufficient to show details at the sub-fm scale.) In the 60 fm range and assuming the classical-electron radius (re =
2.82 fm), the electron and proton potentials are clearly delineated. At 10 fm, the potentials significantly alter each other
and the “effective” charge is less than 1 for either of the particles (the dipole approximation still works). As the (e#p)
pair shrinks further, the electric field of the electron is progressively cancelled by that of the proton and the residual
proton-charge radius is limited to femtometers. The fact that there is any residual charge is attributable to the extra
mass and relativistic nature of the proton’s inner structure.
What do these changes mean to the problem at hand? To first order, we’ll assume that the changes in electron size,
fields, and deBroglie and Compton radii can be ignored. The electron will be considered as a classical charge. We will
treat the proximate electron–proton as a small dipole rather than the large structure that would result from using the
deBroglie or Compton radii. The Heisenberg Uncertainty Principle, as applied to the tightly bound electrons(s), will
be modified by the proximate presence of the proton(s) so that the kinetic energies required to shrink free electrons to
nuclear sizes are no longer in the 100 MeV range.
246

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

Figure 2. (a) Electric potential between, and (b) EM mass-energy distribution of, an electron (assuming a classical radius) and proton separated
by 60, 10, and 0.5 fm. Note change in “size” of both particles as they approach each other and the change in vertical scale between (a) and (b).
We have shown how the Coulomb-barrier problem might be solved with the lochon model and laid a foundation for
the D–D nuclear interaction with the help of tightly bound, highly energetic electron pairs (lochons). Now we further
extend the model to see how it affects the nuclear interaction.

3.3. Nuclear interactions
Examining the traditional D–D to 4He∗ transition (Fig. 3, where ∗ indicates an excited state of the nucleus), we see the
broad transition region (bracketed) beyond 23.8 MeV above the 4He ground state. This “Q” value (broad arrow at 23.8)
is the energy difference that corresponds to the mass difference between the deuterium pair and the 4He atom. Even
with the improbable event of D–D tunneling from zero kinetic-energy states, the excess mass of the deuterium atoms
would put the deuterons into this high level. These excited 4He∗ states are above the fragmentation levels at ∼20.6 and
19.8 MeV (dashed arrows). While fragments from both levels have been observed in LENR experiments, the radiation
fluence levels are not nearly high enough to explain the excess heat observed. Furthermore, the measured concentration
levels of 4He have been orders of magnitude higher than would be expected from standard theory and, in some cases,
have been high enough to account for the observed heat if all excited-state energy (∼24 MeV) is converted to thermal
energy [21]. How could this happen? Since the observed data is not possible within the known framework of nuclear
physics, something else must be going on.
This figure, which shows the excited states of 4He as well as the fragmentation and minimum D–D input energy
levels, indicates that there are no energy levels belowthe 3H +p fragmentation level. Thus, even if a helium nucleus were
to be excited into a heretofore-unobserved meta-stable state below the 20.2 MeV level, it might still fragment rather than
decay back to the 4He ground state. Likewise, in a d–d interaction, if fusion via normal tunneling were to take place, it
would be resonance tunneling into one of the many levels above the broad arrow at 23.8 MeV. Decay from these excited
states would almost always give nearly equal probabilities of fragmentation into the p or n fragmentation channels.

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

247
Figure 3. Energy levels and possible decay branches associated with conventional D–D to 4He∗ fusion reactions, as well as the excited states and
fragmentation and minimum d–D input energy levels of 4He [10]. Note that these values include the mass energy of the atomic electron(s).
However, if the excited state is able to more quickly decay to one of the levels below 23.8 MeV, the fragmentation
probability begins to shift to favor the proton channel. This enhanced decay into the lower excited states could be
an explanation for reduced levels of neutrons for the heat generated. However, it does not explain reduced levels of
energetic protons.
3.4. Reduced energy 4He∗
With the ability of deuterons to be drawn from an appropriate PdD lattice site through the lattice and nuclear-Coulomb
barriers to an adjacent reduced-energy nucleus (Appendix A) of a D− ion, the possibility of a low-energy excited
compound nucleus becomes real. With the lochon (the shrunken-orbit electron pair – as indicated by the superscript
#) rather than with the normal atomic electrons, the excited (but lochon-neutralized) helium-4 nucleus may not have
sufficient energy to fragment. Since gamma decay is highly forbidden, how does 4He∗# shed the excess energy to get
to the 4He ground state? It is proposed that existence of this condition and the subsequent decay process is the basis
for the experimental observations of CMNS.
Depending on the actual energy of the excited (compound) nuclei, the decay process could include fragmentation,
or not. Since there may be no standard resonance states of this compound nucleus, the final tunneling could be into a
range of energy levels. This variability would account for the observations in CMNS of excess heat, in both p–p and d–d
reactions, and the observations (or absence) of tritium, 3He, neutrons, and 4He in the d–d reaction. The unpredictability
of experimental results has been a major problem for CMNS. It now may be a validation of the extended-lochon
model. Furthermore, this proposed mechanism accounts for observed “transmutation,” something that many CMNS
investigators did not accept for a long time.
To see how this “new” process can occur, we must take a closer look at the details behind Fig. 3. If an appropriate
state existed below ∼19 MeV, then any fragmentation would become unlikely. We propose a non-fragmentation
mechanism that does not rely on a new state below 20 MeV. This mechanism of tightly bound electron(s) could shift the
248

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

existing 4He ground and excited nuclear states down and it demonstrates how the fragmentation levels can be shifted
up in energy.

The key to the mechanism is the lochon, which during the collision process attains significant energy (keV to MeV
range); but, being tightly bound in an l = 0 ground state, it does not radiate. If it survives the final tunneling of the d+
through the thin residual D−# (actually d+ plus lochon) Coulomb barrier, it may have attained MeV energies from the
work done at the expense of the D− Coulomb potential energy. Thus, it is very tightly bound and the lochon, the D−
nucleus, and the deuteron all have had mass converted into kinetic and field energy. Since the average lochon orbital
radius is so small, even in a circular orbit, the orbital angular momentum is much less than .h. (so that the l = 1 state
is not available). With the lochon being spin-coupled electrons in a filled s-orbital, there is no spin-flip possible to
provide angular momentum sufficient to generate a photon. Therefore, no photonic radiation is possible and there is no
violation of the uncertainty principle as the lochon orbit and size both shrink during the D−D+ collision.
Once the D+ is inside the D− Coulomb barrier, the situation is different from that of the normal d–d scattering
problem. The normal situation compares the mass difference between 4He and 2D, which is just the same as that
between 4He++ and 2d. In our case, the electrons and protons in the helium nucleus with a tightly bound electron pair
and the free deuterium atoms are different animals. (4He∗ is the excited state with normal atomic electrons, and 4He∗#
is 4He∗ with shrunken-orbit electrons.) The external Coulomb field of these “shrunken-orbit” electrons (a source of
their mass) is nearly zero, and a comparable amount has been subtracted from the proton(s) fields. This mass–energy
equivalent has been converted into kinetic energy [22] and into the interactive-field energies (mutual attractions and
repulsion of the electrons and protons).
The nuclear potential is much different also. The Coulomb barrier between the lochon-altered protons (now electric
dipoles) is almost gone. Thus, they can be much closer together. (In the d–d case, all 4 nucleons can then be closer
together.) Since most of the normal nucleon wavefunction is outside the d-d nuclear well, the depth of the 4He∗#
nuclear potential well with which the nucleons are bound is increased by the loss of Coulomb repulsion and the gain
in time spent in the well (increased wavefunction overlap). As a consequence, the “effective” depth of the nuclear well
is further increased, the ground and excited-state energies become lower, and the energy required to break apart the
excited nucleus is higher. If we look at Fig. 4 (with this information applied to Fig. 3), we see that the whole column
on the left is moved down (the energy levels may split and, depending on the model used to describe the new nucleon -
dipole or light proton, their values will be different) and everything on the right (except for the arrow) is moved up.
The Q arrow at 23.8 MeV moves down with the column; because, while the energy distribution of the 4He# and
4He∗# (which states may never actually exist) is different from that of 4He and 4He∗, the mass difference between 4He#
and 2D#, is still nearly the same (the protons have lost about the same mass that the lochon has gained). Thus, the
deuterons tunnel into nearly the same states that they would have without the energy reduction. However, with the
tightly bound lochon, the states are no longer the same states. And, if they were stable states, they would be shifted
down in energy (along with the ground state if it exists with the lochon).
4. Fragmentation
Do the 4He∗# fragmentation levels really rise above the 23.8 MeV D–D energy level? Well, the elimination of nucleon
Coulomb repulsion is considered to decrease the ground-state level by about 0.73 MeV (but that is based on comparisons
of isomers differing by only one atomic number). If we consider a lochon in the nuclear region, the difference resulting
from the double-negative charge could be 1-2 MeV (the increase in nuclear binding energy, which also raises the
relative fragmentation energies by at least that amount). Since fragmentation energy is a strong function of internucleon
distance, and the lochon has such a great impact on that, this energy could easily go up by another MeV or two.
So the answer is seen in Fig. 4. The lowest “4He∗#++ + lochon” fragmentation level is above not only ∼22.8 MeV, it
may be above the Q# = 23.8 MeV value. The number of fragmentation levels of the lochon-neutralized nucleus may

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

249
Figure 4. Energy levels and possible decay branches associated with the lochon model D+D to 4He∗# fusion reactions and minimum D+D input
energy levels of 4He#. Note that these values include the tightly bound-electron-induced adjustments to the nuclear potential and the mass energy
of the atomic and shrunken-orbit electrons.
be increased because the neutrons and tightly bound electrons can be divided in multiple ways among the two protons
involved. These levels can be considered as representative of centrifugal barriers since it takes increased kinetic energy
and angular momentum to get to them.
This lochon modification of the compound nucleus (4He∗#) energy levels can thus explain the absence (or change
in ratio) of fragmentation in LENR identified as item 2 in the introduction.
5. Alternative Decay Paths
Perhaps important for the reduction of fragmentation is the number of accessible shrunken-orbit nuclear-energy levels
proposed to be now below the fragmentation levels. Any occupied levels above the 3He#, p#, and 3H# fragmentation
levels are transitory (rapidly fragmenting or decaying to these lower-energy levels). In addition to their normal decay
processes (which do not compete well with fragmentation), the excited nucleons may reach lower-energy stable levels
by transferring their kinetic energy to their tightly bound electrons. These electrons couple this energy to the nearby
atomic electrons or the lattice (via near-field radiation, phonon, and plasmon interactions). In the presence of this
new decay process, normal decay processes from excited-nuclear levels 4He∗# to the fragmentation or lower levels is
inhibited and becomes a low-probability process.
An important point to note is that the new energy-transfer process to and through the tightly bound electrons is not
rapid. It is steady. But, even for states above fragmentation levels, this initial decay process may compete well against
fragmentation. Furthermore, the presence of the tightly bound electron(s) interferes with “normal” decay processes that
depend on stable resonance states (e.g., gamma decay) and/or angular momentum (e.g., fragmentation). The steady
process means that the total time for decay to ground is long, relative to normal decay modes. However, it “drains”
EM field energy necessary for the formation of gamma rays and utilizes the angular momentum that would allow
fragmentation. While it is slowing these processes, it lowers the nuclear energy below the fragmentation levels and
interferes with the stability of the lower nuclear states shown in Fig. 4. Thus, as long as the tightly bound electrons are
present, the helium nucleus is not in a normal state and the indicated excited levels are only representative and cannot
be considered real.

250

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

The presence of a lochon so-greatly reduces the Coulomb barrier to fusion, that non-zero-angular-momentum
4He#∗ states might become accessible. These states are more accessible via shrunken-orbit electrons and their decay
through normal processes (e.g., gamma decay to lower levels, or to the ground state) could more readily compete
with fragmentation. Nevertheless, it is unlikely that gamma decay from such states (or direct tunneling to the stablefragments)
could compete with the energy decay process from proximity coupling through, or in the presence of, the
tightly bound electron(s) or lochon.
During the p–p or d–d lochon-enhanced fusion process, the lochon could divide or perhaps attain energies in theMeV
range from coupling with the protons accelerating in the nuclear potential. These rapidly accelerating and decelerating
electrons would then radiate Bremsstrahlung or would proximity couple their energy to the adjacent Pd electrons [23].
This extended-collision/radiation process lasts:

1. Until the neutral entity (2He∗# or 4He∗#) drifts into a neighboring nucleus in a transmutation process;

2. Until one (or more) of the shrunken-orbit electrons combines with a proton (p + e ⇒ n + υ, via the p–e–p,
p–2e–p, etc. reactions); or,

3. Until ejection of the energetic electron(s) from the nucleus and the (D or 4He) ground state is reached.
Thus, the last of the introductory arguments against cold-fusion have been addressed.

6. Conclusion

The Lochon Model provides a basis for penetration of the nuclear Coulomb barrier in low-energy p–p and d–d fusion
reactions. Depending on the actual energy of the excited (compound) nuclei and the number of tightly bound electrons,
e#, still attached (0, 1, or 2) after the nuclear Coulomb barrier has been penetrated, the decay process could include
fragmentation, or not. This extension of the model accounts for the observations in CMNS of excess heat, in both p–p
and d–d reactions. It also explains the observations (or their absence) of tritium, 3He, neutrons, and 4He in the d–d
reaction. The ability of the tightly bound lochon - to alter energy levels in the nuclear potential wells and fragmentation
energies of the excited 2H#∗ and 4He#∗ atoms - permits decay to the 2H or the 4He ground states or allows sufficient
time as a shrunken-orbit atom or neutral nucleus for transmutation of lattice atoms or impurities.
Variation of observed results of LENR is thus based on the number of tightly bound, energetic electrons remaining
within the nuclear region after initial D−D+ fusion and during the subsequent decay process. The Extended Lochon
Model, in its present form, may explain all observed LENR processes (model-predicted levels and observability of
Bremsstrahlung and any neutrinos must still be determined), but not necessarily the best means of, or materials for,
producing them. That comes next.

Acknowledgments
This work is supported in part by HiPi Consulting, New Market, MD, USA; by the Science for Humanity Trust,
Bangalore 560094, India; by the Science for Humanity Trust Inc, Tucker, GA, USA; and by the Indian National Science
Academy.
Appendix A: Development of Electron Coupling into Tight Orbits

For the reasons stated above and in our earlier work, the D−D+ pairs are formed within the loaded PdD lattice (or
interface and defect regions). This phonon-induced charge polarization is a maximum during the closest encounter of
the deuteron ions. At this point, the monopole–monopole interaction is highest and the resulting E field can exceed the
phonon field, to strip one or both electrons from the D− ion. This exchange thus forms molecular deuterium (perhaps
only temporarily, if the lattice barrier separates the molecule rather than drawing it into one lattice site).

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

251

This appendix addresses the electron energies and their exchanges assuming that the electrons are maintained as
a pair long enough to move too deep into the D− potential well to be removed by the D+ E field until after Coulomb
barrier penetration. The problem is addressed as a monopole–monopole interaction with the deepening electron energy
levels treated as a modified Stark Effect. (The electron-energy levels in the D− ion deepen in the E fields produced by
the lattice and then by the D+ ion.)
Of importance to the lochon model is the fact that the electrons in the D− do work and, thereby, move deeper into
the Coulomb potential well. Therefore, we need to compare the change in potential between the D− and D+ ions
(_Vdd) relative to the work done in bringing them closer together (from l1 to l2) by a small distance, _l = l1 − l2.
In the simplest model, for the conditions of a D− D+ Coulomb attraction much greater than phonon effects and for a
dielectric constant of ε,
1. Change in potential: _Vdd = −e2(1/l1 − 1/l2)/ε =∼ (e2/ε(l1l2))_l. (Thus, for small _l, I1 =∼ l2 =∼
l => _Vdd =∼ (e2/εl2)_l.)
2. The force between the ions: Fdd = −dVdd/dl = −e2/εl2.
3. Work by each deuteron ion: _W1 = _W2 = Fdd ∗ _l/2 =∼ (−e2/εl2)(_l/2).
The _l /2 term comes from the near-equal masses of the D− and D+. The work done by the D− electrons equals
that done by the D+ ion. Each ion moves the other by 1
2 the change in total separation distance _l. The total work of
the D−D+ system (_Edd = _W1 + _W2) is approximately the potential difference between l1 and l2. We can look
further into the conservation of energy to see where the work comes from. The D+ ion does work by converting some
of its field energy into kinetic energy (and in overcoming the lattice barrier early in the process). The D− ion does the
same; but, what field energy is converted? It must be the negative portion.
The lochon provides the negative charge and therefore the D− ion portion of the work and field energy to bring the
deuterons together and to give them kinetic energy to overcome the lattice barrier. In providing this energy, the lochon
drops deeper into the D− potential well and gains kinetic energy. These distances of the changing lochon orbits (charge
= −2e, mass = 2 electron masses) relative to the D− nucleus equal r1 and r2 with r1 > r2. Things are different from
above because we are now looking at many electron orbits rather than only a portion of a single phonon cycle. The D−
nucleus does not significantly change its KE relative to the lochon during a decay of the lochon orbit. Therefore the
lochon does almost no work on its deuteron nucleus in the D− center-of-mass system. (It does not move the deuteron
within this system; but, it moves the whole D− relative to the D+.) However, the nucleus does work on the lochon.
The lochon gains _Vdl/2 in KE from the orbit decay and (from the virial theorem) can do the same amount of work on
the D−D+ ion pair. Again, assuming that the D−D+ interaction energy greatly exceeds that of the lattice barrier:
4. _ in potential (D− nucleus & lochon): _Vdl = −2e2(1/r1 − 1/r2)/ε = 2e2/(r1r2) ∗ _r.
5. Work done in decay of D− electrons: _Wdl = _Vdl/2 =∼ (e2/εr2)_r.
Assuming that the model holds, and if _Vdl/2 = _W1 = _Vdd/2 (e.g., if _r/r2 = _l/2l2) and l > 2r, then
_l > 8_r. Thus, unless early in the process the lochon has moved deeply into its potential well, the inter-deuteron
distance rapidly shrinks to the lochon orbit radius [24] (l = r) and will share the bound electrons. Sharing the electrons
(lochon) between the deuterons will increase the size of the electron distribution and eliminate the D−D+ Coulomb
attraction. If the electron orbit has shrunk prior to the transition fromD−D+ to D–D, the nowtightly bound electrons still
provide better screening than that from free electrons or from normal molecular electrons. Furthermore, the momentum
of the deuterons will continue to close the deuteron gap and thereby further shrink the electrons’ (now molecular)
orbit. The later the sharing of electrons, the deeper the electrons will be in their potential well, the higher the deuteron
momenta, and the smaller the Coulomb barrier that must be tunneled through. Therefore, the phonon-induced collision
process at the lattice barrier is the starting point that can lead to, or block, the proposed extended-lochon model.

252

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

The deuterium sites in PdD are highly confined by the Pd lattice and screened from each other by the Pd-lattice
electrons. Thus, the initial conditions are not amenable to any simple analysis. Since, work done by the bound-electron
pair in overcoming the lattice barrier may be critical to the model; it must be addressed in a more sophisticated manner
than is possible in this paper.
As described here, the lochon motion to deeper regions of the D− potential well (i.e., to tighter orbits with increased
kinetic energy) can proceed without any radiation. Nevertheless, mass energy of both deuterons is lost (Appendix B).
The process of shifting between the normal D− ion in a lattice site to a “reduced-energy-nucleus” ion at the lattice
barrier is reversible (unless, or until, the deuteron nuclear potentials overlap) and can continue to oscillate at the phonon
frequency. However, with sufficient deuteron and boson energies within the Coulomb wells, energetic-lochon-catalyzed
fusion may occur as described in the text. Alternatively, the “shrunken” electron orbits at the point near deuteron collision
may allow closer approach of the D−D+ pair and the resultant stronger coupling would permit the small D+ ion to be
drawn into the D− ion’s lattice site. The now-allowed proximity of the deuterons, one perhaps with electrons in the
metastable nought orbit (Appendix C), can thereby provide boson-catalyzed fusion (similar to muon-catalysed fusion).
Appendix B: Electron Size in the Nuclear Regions
This appendix addresses the “size” of an electron as it must be pictured in its interaction with protons at nuclear distances.
It is based on the presently-accepted model that the mass of the electron is totally electromagnetic [25]. Furthermore,
the electrostatic field defining the charge is assumed to be the dominant EM component. The proton’s external EM
field is identical to that of the electron. Therefore, if size is based on charge field alone, the electron and proton would
be the same size. However, since the Compton radius is based on total mass and proton mass from the charge field is
only a very small percentage of the proton mass energy, this useful radius is much smaller for the proton than is that
for the electron. On the other hand, when the electron and proton are close together, other things come into play. Of
major importance is the “collapse” of electron size as its far-field component is cancelled by that of the proton and the
long-range monopole potential becomes only the short-range potential of a dipole (Fig. 2a). Other things happen as
the electron and proton approach one another.
The additional kinetic energy of tightly bound electrons e# means that an electron “size,” based on the deBroglie
radius (λdB/2π), shrinks as λdB = h/mv. While this is actually related to the time-averaged electron-charge distribution
in an orbit, not any actual electron size itself, there is still a frequency associated with the orbit and thus the deBroglie
wavelength. Since frequency is related to energy and energies are additive, the deBroglie frequency (related to the
deBroglie wavelength, νdB = v/λdB) is added to the Compton frequency (related to the Compton wavelength, νC =
c/λC) and the summation provides a higher frequency (hence energy: Et = h(ν1 + ν2)) that gives a smaller electron
radius. (This shrinking is a basis for association with the momentum/size relationship of the Heisenberg Uncertainty
Principle.)
A poor understanding of the various relationships between electron size and time-averaged charge distribution is
a common source of some miscalculations of Coulomb screening by electrons. For example, tunneling is considered
to be instantaneous. Therefore, screening by an electron “cloud” is not an average value, but a statistical probability
of finding an electron at a given location at the instant of tunneling. This appendix provides some further insight into
different usages of electron sizes.
If the much larger Compton electron radius is assumed, rather than the classical radius, one might think that the
change in electron shape and effective charge begins much further from the proton. However, in Fig. 2a, because the
plotted potential is a 1/r function (and the total energy and charge is fixed), the actual amplitude and shape of the
exterior electron field cannot change with the model. The difference must be in the definition, not in the particle. With a
Compton radius of 384 fm (0.384 pm), the statement would then be that the electron’s “size” extends much further to the
extremities of the field than does the classical-electron radius of 2.82 fm. If the potential “inside” the chosen electron

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

253
radius is different, then the field in that region will change and the magnitude of the total field must be renormalized to
provide the total mass/field energy of the electron.
Assuming that the electron mass is entirely electromagnetic in origin and that the field-energy density is proportional
to the field squared, then the mass-distribution of the electron can be pictured as much more localized than that of the
potential distribution (Fig. 2b). (Field E is proportional to the derivative of the potential, giving 1/r2 dependence.
This implies that energy density E, proportional to E squared, varies as 1/r4.) The external-EM portion of the proton
energy can be pictured in a similar manner. (Remember that this charge-field component is only about 0.05% of the
total proton mass.) Because of the great increase in localized mass density relative to the potential, Fig. 2b is shown as
a semi-log plot of the non-relativistic charge-field energy only. If the proton’s mass energy (held to be relativistic-field
energy by one author -AM) were included in the figure, it would extend 3 orders of magnitude above the curves shown.
Expanding the resolution of the electron EM mass profile (not shown), one could see that the classical radius includes
>99% of the mass energy. The Compton radius of the electron extends to some very-low value of field or potential.
The electric potential at the 0.15 fm Compton radius of the proton is still very high (Fig. 2a).
Assuming that charge particles are not singularities, the electric potential must be finite and must reverse at the
center of the charges. Therefore the field-energy density goes to zero at this point. However, as the electron approaches
the proton at 10 fm distance in Fig. 2b, this electric-field density (mass) of the electron disappears. The electron may
no longer have an identity. Nevertheless, when the electron gets close enough to the proton to eliminate any sign of the
electron’s negative charge, there is still a residual positive charge of the proton. The difference in near-field “charge”
between the electron and proton is a relativistic effect. This is a result of the need to have the 1/r potential continue to
a smaller radius for the proton than for the electron. It takes energy to do this.
The figures described above are for localized charges. They indicate that, when the electron is within Fermis of the
proton, its field-energy density disappears. Where does it come from and where does it go? It comes from the proton
and electron electrostatic fields and goes into motion of the altered and residual field energy (mass and/or charge).
Unless energy is radiated away, or is transferred in some other form, it is bound to the “compound structure” and acts
as energy-equivalent mass. However, the moments of inertia, magnetic moments, etc. of the structures are altered and
energy can be stored in the new angular motion. For an isolated electron–proton structure, this has little consequence
(also it is an extremely short-lived condition - a single transit by the electron?) However, for colliding p+p− or D+D−
structures (where the closely bound electrons may exist for hundreds of orbits or more), it means that the nucleon
wave-functions are altered.

Appendix C: Nought-orbits (n = 0)

In 2005, Jan Naudts posted a paper [19] on the arXiv entitled “On the Hydrino State of the Relativistic Hydrogen
Atom.” He showed that there was a solution to the Klein-Gordon (K-G) equation that is generally rejected as being
non-physical. This solution adds a new atomic orbital at close to mc2. Since the K-G equation pertains to Bosons,
not Fermions, Naudts suggested that the question of square integrability of the Dirac equation (which blocks Fermions
from that level) might be the non-physical requirement instead and thus the K-G solution could be used to support such
a level for Fermions as well. Two papers [26,27] have rejected both suggestions; but, a third [28] has accepted the K-G
solution as real.
Applying this information to our model and assuming the additional K-G solution to be valid (at least for bosons),
the reality of the bound electron pair (the lochon) as a boson means that this level is available as a physically real lowerenergy
state (the n = 0 or nought-orbital). This level will not be explored further here (such as the effects of a doubly
charged boson on the solution). However, neither the n = 1 ground state nor the n = 0 nought orbit has sufficient angular
momentum to permit photonic-energy transfer. Therefore, it is still a forbidden photonic transition. Nevertheless, as a
viable, tightly bound, relativistic-electron state, the nought level can be reached by the model described in this paper
254

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

and it provides additional basis for the extended-lochon theory of low-energy nuclear reactions. This could be a critical
point for both the Coulomb barrier penetration and the energy level at which the H–H or D–D fusion begins.
A potential importance of this nought orbit is the fact that it provides an intermediate stage for fusion via a mode that
is well-known physics - muon-catalysed fusion. Since the n = 0 electron-orbit radius is∼400 fm (at∼507 keV), n = 0
hydrogen would be smaller than muonic hydrogen. But, just as a filled orbital H− ion is larger than the neutral atom,
the lochonic n = 0 hydrogen ion would be larger than a neutral nought-orbit atom. On the other hand, the nought-orbit
molecule (H+ + H−
, or H#2 ) might be smaller than the muonic hydrogen molecular ion (and perhaps ionically bound
rather than covalently bound). Details must be presented elsewhere; but, the implications for nought-orbit hydrogen and
molecules for lattice mobility, lattice-site double occupancy, and muon-catalysed-type fusion are immense. Whether
this is an unreal, a competitive, an incidental, or an assisting process is still to be determined.

References

[1] Y. Iwamura et al., Transmutation Reactions Induced by D2 Gas Permeation Through Complexes (P/CaO/Pd), 14th Int. Conf.
on Cold Fusion (ICCF),Washington, DC, 10 August 2008; andY. Iwamura, M. Sakano, T. Itoh, Jpn. J. Appl. Phys. 41 (2002)
4642–4648.
[2] K.P. Sinha, A. Meulenberg, Laser stimulation of low-energy nuclear reactions in deuterated palladium , Current Sci. 91 (2006)
907, arXiv:cond-mat/0603213, accessed 28 June 2010.
[3] K.P. Sinha, A. Meulenberg, Lochon catalyzed D–D fusion in deuterated palladium in the solid state, Nat. Acad. of Sc. (India)
Letters 30(7&8) (2007), arXiv:0705.0595v1 accessed 28 June 2010.
[4] K.P. Sinha, A. Meulenberg, A model for enhanced fusion reaction in a solid matrix of metal deuterides,
ICCF-14, International Conference on Condensed Matter Nuclear Science. Washington, DC, 2008. http://www.lenrcanr.
org/acrobat/SinhaKPamodelfore.pdf.
[5] A. Meulenberg, K.P. Sinha, Tunneling beneath the 4He∗ fragmentation Energy, ICCF-15, International Conf. on Condensed
Matter Nuclear Science, Rome, Italy, 2009.
[6] P.W. Anderson, Phys. Rev. Lett. 34 (1975) 953.
[7] K.P. Sinha, Infinite Energy 29 (2000) 54.
[8] No attempt is made to describe the electron orbits of a two-electron atom in a lattice. This is difficult enough for such an atom
in free space [e.g., G. Tanner, K. Richter, J. M. Rost, Rev. Mod. Phys. 72 (2000) 497–544 ].We know the orbits exist, but not
what they look like.We have assumed a quadrupole, rather than a dipole configuration.
[9] Presence of D− ions and D− D+ pairs in the loaded-PdD lattice has not yet been sought or confirmed.
[10] A. Krauss, H.W. Becker, H.P. Trautvetter, C. Rolfs, K. Brand, Nuc. Phys. A 465 (1) 150–172.
[11] Useful definitions of terms in the paper that are not familiar to all readers may be accessed on the
Wikipedia. http://en.wikipedia.org/wiki/Muon-catalyzed_fusion#Process .http://en.wikipedia.org/wiki/Born-Oppenheimer;
http://en.wikipedia.org/wiki/Phonon_drag;
http://en.wikipedia.org/wiki/Adiabatic_theorem; http://en.wikipedia.org/wiki/Mass_deficit;
[12] A. Takahashi, N. Yabuuchi, ICCF-13 International Conf. on Condensed Matter Nuclear Science, June ’07, Sochi, Russia.
http://www.lenr-anr.org/acrobat/TakahashiAdeuteronst.pdf.
[13] S.H. Patil, J. Chem. Phys. 118 (2003) 2197.
[14] Tom Barnard, Private Communication, & http://www.ichaphysics.com/.
[15] C. Kittel, Introduction to Solid State Physics, 7th Edition., JohnWiley and Sons Inc., 1996.
[16] The proposed existence of D− D+ pairs in a lattice is based on accepted physics [6]. Mechanisms proposed for electrons and
their ground state moving deeper into an atomic or molecular potential well are seldom discussed.
[17] F. Raiola et al., Eur. Phys. J. A 19 (2004) 283–287 and A. Huke et al., Eur. Phys. J. A 35 (2008) 243–252.
[18] Electron mass is considered to be entirely from electromagnetic-field energy. Therefore, the electric-field energy of the proton
must also be mass-equivalent energy.
[19] J. Naudts, On the hydrino state of the relativistic hydrogen atom. http://arxiv.org/abs/physics/0507193 accessed 28 June 2010.
[20] For an electron, the total energy = Eo = rest mass − binding energy = ∼ αmoc2 =∼ (1/137)511 keV = ∼ 3.7 keV => binding

A. Meulenberg and K.P. Sinha / Journal of Condensed Matter Nuclear Science 4 (2011) 241–255

255
energy = ∼507 keV. For a lochon, the binding energy = ∼1 MeV.
[21] M. Mckubre, F. Tanzella, P. Tripodi, P.L., Hagelstein, Proc. of ICCF8, 70, 3 (2000).
[22] Treatment of the kinetic energy of a reduced-field, relativistic, electron is beyond the scope here.
[23] K.P. Sinha, A. Meulenberg, Radiative-field quantum-coupling between closely-spaced surfaces. http://arxiv.org/abs/0902.0682
accessed 28 June 2010.
[24] These simplified calculations assume circular electron orbits. No corrections have been made for the deepening of the orbit as
the system approaches a 1-dimensional geometry and for the stark effect. On the other hand, neither is there a correction for
the stretching of the electron orbit toward the D+, from proximity effects of the D− D+ potential well or for the linear electron
orbit that makes rmax of the linear orbit greater than ravg of the circular orbit.
[25] E. Fermi, Correction of a Contradiction between Electrodynamic and Relativistic Electromagnetic Mass Theories, Nuovo
Cimento 25, 159–170 (1923). Translation at: http://www34.homepage.villanova.edu/robert.jantzen/mg/fermi/fermi4c.pdf
[26] N. Dombey, Phys. Lett. A 360 (2006) 62.
[27] A.S. de Castro, Phys. Lett. A 369 (2007) 380.
[28] T. Nadareishvili, A. Khelashvili, Some problems of self-adjoint extension in the Schrodinger equation.
http://arxiv.org/abs/0903.0234 accessed 28 June 2010.…but the solution (b.2) again satisfies (2.1) in the (2.10) and therefore
the hydrino (additional) states must be retained.

being tightly bound in an l = 0 ground state,

"l" is not the number one but the letter lower case "l" attempting to refer to quantum mechanical angular momentum state. Now this is nonsense because l is not a quantum mechanical observable. The QM observables are Jz or J^2=j*(j+1), and since we are talking about an electron with spin 1/2 there is no l=0 state. The lowest angular momentum state can be j=1/2 corresponding to a linear combination of l=0 and l=1 states with the spin 1/2 of the electron.

So even where they lost you this is nonsense, but that is not really the point, here.

the lochon's lowering effect on the Coulomb barrier supposedly enables not only deuterium-deuterium, but also proton-proton interaction. And, I follow their claim that, since no resulting gamma radiation is detected, the released energy is emitted as heat

But this is all BS. The lochon cannot exist because its properties (tightly localized electron density providing effective charge screening) violates the Heisenburg uncertainty principle. It also violates the Pauli principle (Fermi-Dirac statistics) since only electrons with energies above the Fermi energy can participate in this kind of screening. We already have an example of electron phonon interactions producing localized states. It is called the cooper pairing of superconductors. Properly taking account of the strength of electron phonon coupling and the fact that the pairing can only occur among electrons with energies near the Fermi-edge produces coherence lengths of the order of 3000 Angstroms (1000 lattice spaces not 1/10th of a lattice space as this lochon theory requires). [See Mourakchine http://arxiv.org/PS_cache/cond-mat/pdf/0405/0405602v1.pdf].

Now you might argue well who is to be believed. The theory of superconductivity is well established, experimentally well studied and theoretically well explained. It has all been worked out in very great detail using physical laws and principles that are broadly accepted.

None of the gibberish that he writes is accepted by any competent physicist.

And there was no peer review, since any competent solid state physicist, and I am not a solid state physicist, would have pointed out these issues and many many more.

Then there is all the nuclear physics nonsense,since no resulting gamma radiation is detected, the released energy is emitted as heat

But there is no way that nuclear processes (scale lengths of a fermi - 10-15cm couples to lattice phonons directly - scale lengths of 10-8 cm). It is like rocking a battleship with pond ripples. The frequencies are incommensurate by the same number of orders of magnitude, and the energy does not work either. You cannot put 2Mev of energy into a phonon or any finite number of phonons.

And then there is the fact that you cannot even get such a nuclear transition in the first place because of the laws of nuclear physics.

The simple underlying problem is that these guys are trying to explain a phenomenon that have never been consistently and repeatedly demonstrated with a claimed set of processes that violate every principle of physics known. These are, as another critic stated, extraordinary claims. Acceptance will require extraordinary proof. Such extraordinary, convincing proof has not been forthcoming.

Naysayers are allowed to remain skeptical until there is overwhelming evidence to the contrary.

There are supposed to be standards of independence, competence and intellectual honesty.
***01101000 01101111 01110010 01110011 01100101 00100000 01110011 01101000 01101001 01110100 00001101 00001010
Fun Binary Converter < http://www.paulschou.com/tools/xlate/ >
Loads and loads of it. The Peer Review process was corrupted by the hot fusion boys, and the intellectually and dishonestly drove Pons & Fleischmann out of town.

What these guys are doing is similar to what the Global Climate Change guys did.
***And the hot fusion boys. You can thank them for the current state of “science” — spending $billions on 6MJoules of energy while this technology has generated hundreds of times that at a fraction of the cost.

They set up a peer review system of insiders with a point to prove and that is what they insure gets published.
***You’re the one hung up on peer review. These guys are their peers. There is no such thing as a peer review when the science is new, we have all seen that happen with the hot fusion boys.

You may think that that is the general rule in the sciences, but it is not.
***It is the general rule in this science. Due to the hot fusion boys having someone invade their turf.

The American Physical Society maintains pretty high standards and when folks try to manipulate it, resignations happen, and resignations by people who have the respect of the general physics community.
***Then read up on the American Chemical Society compendiums in LENR.

None of the guys on the editorial board are folks that anyone would regard as just good solid capable physicists.
***I regard them as that. I regard the author as a solid capable physicist. Basically, the author and the peers engaged in review of this document all have higher capability as physicists than me or you.

I regard them as that. I regard the author as a solid capable physicist.

Well, he has written down theoretical claims that violate most of the known laws of electrodynamics, quantum mechanics and nuclear phenomonology with no stated justification. That does not make him a solid and capable scientist. I have no problem with someone claiming to discover a new principle of physics. It just has to be based upon a sound understanding of sound experimental evidence. For instance, if Sinha wants to disprove the Heisenberg uncertainty principle he is welcome to try. Show some data and explain why this data is at variance with all the other data on the subject. But you don't just trip over it ignorant of the fact that it is even there and claim you are doing physics.

And the hot fusion boys. You can thank them for the current state of “science” — spending $billions on 6MJoules of energy while this technology has generated hundreds of times that at a fraction of the cost

You have not been paying attention, have you. Your guys have not generated gigajoules of energy. A gigajoule is the equivalent of a ton of TNT. These guys have not individually or collectively detonated a ton of TNT. They have not even released a pound of it. We would all know it if they did.

Second, hot fusion does work. We have a picture of it working. It is called Operation Greenhouse George.

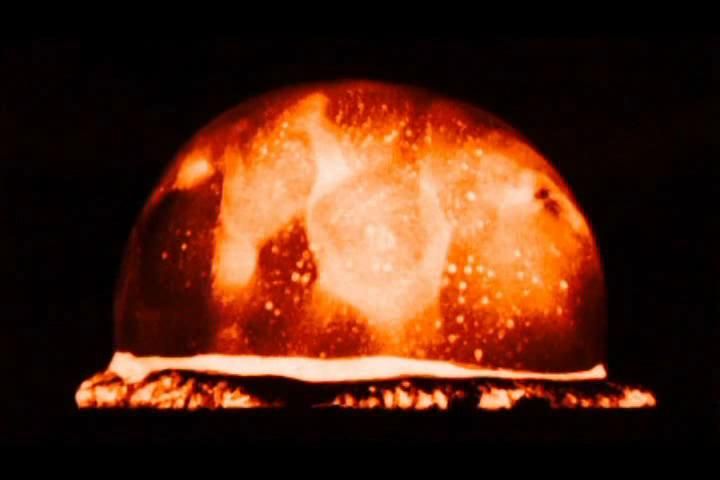
And by the way, they are not claiming that the laws of physics stopped working and that you cannot detect the heat or light or radiation. Nor has anyone accused them of making claims that violate laws of physics.

AndyJackson said: "The simple underlying problem is that these guys are trying to explain phenomenon that have never been consistently and repeatedly demonstrated ..."

That's my view. Couldn't say it better.

Those who have convinced themselves that "cold fusion" exists have done so, I believe, because it might conceivably change dramatically the dependence of the U.S. on conventional sources of energy.

Similarly, those who convince themselves that man is contributing to harmful global warming do so because it justifies massive intrusion into our economy.

Neither of these efforts has much to do with science, except to cast science and scientists in a poor light.

the American Physical Society has been right in the thick of promoting AGW and diddling the peer review process.

This is exactly backwards. A former president of the APS, Cherry Murray along with some members have tried to do this. It led to a rebellion of a very distinguished cadre of members of the APS who pointed out, quite publicly, the fraud that was being committed. Murray's response was to try to investigate who had leaked the email list. Her year's term ended with her being regarded as something of a disgrace.

Most members of the APS are agnostic on the subject of GCC, believing that the issue is certainly worth study, meaning developing better physical models and computer simulations and integrating all the available sensors (land based, oceanographic, satellites, etc) to see if we can figure out if anything is going on at all.

The Peer Review process was corrupted by the hot fusion boys, and the intellectually and dishonestly drove Pons & Fleischmann out of town.

The biggest critics are not the hot fusion community, whose principle issues are trying to confine hot plasmas for long enough to get fusion to work at laboratory scales. What the cold fusion guys do or claim to do is simply irrelevant. The biggest critics are in the nuclear physics community because the LENR guys have made claims that counter everything that the nuclear physics community knows from theory and experiment, but the LENR crowd have measured nothing and written no explanation that suggests that what the nuclear physicists understand is in error. All the cold fusion guys do is accuse the nuclear physics guys of being closed minded.

But with folks like Sinha the biggest critics are about to become the solid state physicists. As I have already indicated this stuff is complete and utter nonsense.

If it all worked so well as its advocates claim, then put one of these power producing cold fusion cells in an electric car and take a few turns around the block. It will convince everyone.

Well, he has written down theoretical claims that violate most of the known laws of electrodynamics, quantum mechanics and nuclear phenomonology with no stated justification.
***The justification is the 14,000 times the excess heat Pons-Fleishmann effect has been replicated. If you do not accept that as an observational point, then you have no need to bother with digging down into the physics as KP Sinha does.

That does not make him a solid and capable scientist.
***What does make him a solid and capable scientist is all the other peer reviewed publications and what he does for a living.

I have no problem with someone claiming to discover a new principle of physics. It just has to be based upon a sound understanding of sound experimental evidence.
***Like I said, the PF effect is replicated 14,000 times. That is the experimental evidence. Sinha’s theory explains the evidence.

For instance, if Sinha wants to disprove the Heisenberg uncertainty principle he is welcome to try.
***If there is some observation that disproves it then it is legitimate to use it in a theoretical approach. The way Richard Feynman won his Nobel prize was by disregarding an accepted principle, and later on he was vindicated.

Show some data and explain why this data is at variance with all the other data on the subject.
***Start here for the data.
http://www.lenr-canr.org/FilesByDate.htm

But you don’t just trip over it ignorant of the fact that it is even there and claim you are doing physics.
***Good advice, that is, if you have been over the same data that Sinha has.

You have not been paying attention, have you. Your guys have not generated gigajoules of energy.
***http://www.pdfdocspace.com/docs/47627/cold-fusion-and-the-future.html

A typical cold fusion experiment using Seebeck calorimeter
costs roughly $50,000 including all equipment. Some have produced 50 to 300 megajoules in one run. They have achieved the two goals hot fusion has failed to reach for 60 years: breakeven and full ignition.

The Tokamak Fusion Test Reactor (TFTR) at the Princeton University Plasma Physics Laboratory, U.S. Department of Energy cost “about a billion dollars” to construct and $70 million a year to operate. It produced 6 megajoules in one experiment, the world record run for hot fusion.

A gigajoule is the equivalent of a ton of TNT. These guys have not individually or collectively detonated a ton of TNT.
***Yes they have.

They have not even released a pound of it. We would all know it if they did.
***Your assumption seems to be that much energy being released in one instant. Your assumption is invalid.

Second, hot fusion does work. We have a picture of it working. It is called Operation Greenhouse George.
***It works as a bomb, an uncontrolled release of energy. For the last 60 years we have tried to harness that huge release of energy, and the best controlled run that a $10B Tokomak reactor has attained is 6MJoules. What else have we gotten for all that effort, do we have hot-fusion cars or airplanes or water heaters? No. Your analogy of saying that fusion works because we have a fusion bomb is like saying that there’s plenty of water to drink because we have oceans.

Disclaimer: Opinions posted on Free Republic are those of the individual posters and do not necessarily represent the opinion of Free Republic or its management. All materials posted herein are protected by copyright law and the exemption for fair use of copyrighted works.