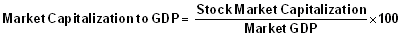My comment: "Very interesting. Theoretically, the value (capitalization to GDP ratio) should be around 100%, not 50%. That says that historically, the stock market has been under invested."
Refering to this formula:
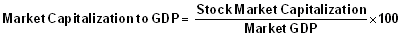
Your comment: "What's the theory here? Could you expand?"
Sure. The GDP of the stock market would be all the income of the all the stocks in the market for one year. The market capitalization would be all the prices of all the stocks times all the shares.
I thought market GDP might refer to the capitalization to the market's revenue, but the definitions I have seen don't seem to support that.
A ratio of 1:1 of Price to Earnings would be very low for the market. I think I must be misunderstanding the definition some way. I cannot see my error. Can you?
I think your analysis is exactly right --- up until the conclusion.
Think of a given stock market as a stock of a single company. The price of it today is the market capitalization. The earnings of the "company" that issued the "stock" is "the market GDP" (whether trailing or projected is not important at the moment, right?). The ratio, as you correctly pointed out is simply the market's P/E.
In theory, then, what should this P/E equal to? This is clearly an ill-posed question, because P/E of any stock reflects not only the current or next-period earnings but also (the current beliefs regarding) all future earnings (in periods 1,2,...infinity). That is why we see P/E values having a wide range of values: two companies with the same E are assigned by the market very different P's because people have widely different beliefs about the future of the two companies. We also see that P/E of a given stock changes over the course of a quarter, although the reported E stays the same: people change their beliefs depending on the news they acquire, and assign P accordingly.
It appears, therefore, that the "theory" cannot assign the value of P/E to the market just as it cannot do that for any stock of a real company: other factors (beliefs) must be taken into consideration by any theory that attempts to do so.
[ Consider the "market" in the formula to be the set of SP500. Then the formula yields a widely reported "P/E of the SP500." As you know, people often speculate on whether the market is under- or overvalued depending on the value of that P/E. This P/E, in line with the foregoing, is also oscillating --- from low teens, as far as I remember to 35 or so.]
Does all this make sense to you?